EE1.el3:  Exercise solutions

A. Physical quantities

1. How many tonnes of water are there in an Olympic size swimming pool?
   2. 10³ tonne
      1 tonne = 1 m³, pool 50 × 2 × 20
2. What is the mass of a grain of granulated sugar?
   2. 10^-9 kg
      (0.5 × 10^-3)^-3 × 4 = 0.5 × 10^-9
3. A young ostrich that weighs 100 kg is travelling at 54 km/h. Linear momentum is defined as p = mv where m is the animal's mass and v is its velocity.
   1. What is the magnitude of v in SI units?
      v = 54 × 10³ / 3600 ms^-1
   2. What is its momentum?
      p = mv = 100v
   3. What are the dimensions of p?
      M L T^-1

1. The Fresnel number is used describe diffraction of electromagnetic waves:
   

   F   =   ax / D λ

   where a is the radius of the aperture, D is the distance from aperture to screen and λ is the wavelength.
   1. What are the dimensions of a, D and λ?
      L, L and L respectively
   2. Hence, what is the value of x required to produce a dimensionless quantity?
      x = 2
   3. So, in order to perform an experiment at the same Fresenel number using light with twice the initial frequency (i.e., half the wavelength), what change must be made to the size of the aperture? (Hint: you may assume that the aperture-screen distance remains unchanged.)
      1 / √2

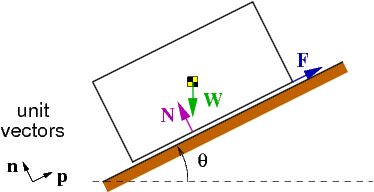
1. Calculate force F in terms of the unit vectors i and j for a block on a slope, as in Fig. 1 (left, and in lecture slide C.13), given the relations:

   W	=	-mg j
   F	=	Fcosθ i + Fsinθ j
   N	=	-Nsinθ i + Ncosθ j

   where θ is the angle between the plane of the slope and horizontal.
   In the j direction: -mg + N cos θ + F sin θ = 0
   In the i direction: -N sin θ + F cos θ = 0
   ⇒   N = F cos θ / sin θ
   ⇒   F ( cos² θ / sin θ ) + F sin θ = mg
   ⇒   F ( cos² θ + sin² θ ) = F = mg sin θ
   ⇒   F = mg sin θcos θi + mg sin² θj

Fig. 1.1: Block on a slope with unit vectors i and j (left), and p and n (right).




2. Calculate force F in terms of the unit vectors p and n for a block on a slope, as in Fig. 1.1 (right).
   In the n direction: N = N n
   In the p direction: F = F p
   and  W = W sin θ p + W cos θ n
   Resolving components in the p direction, we get: F = W sin θ = mg sin θ
   ⇒   F = mg sin θcos θi + mg sin² θj

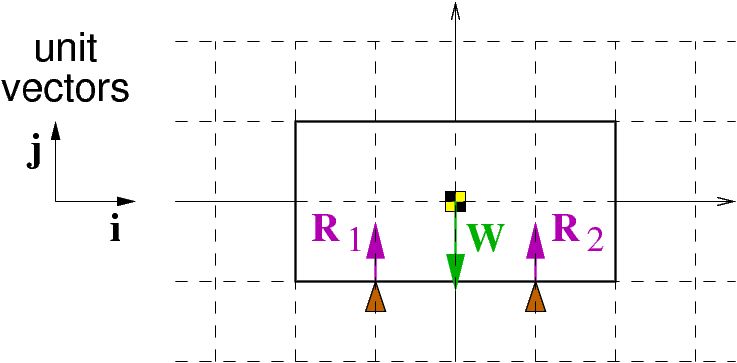
1. A 4m-by-2m box is at rest on two simple supports, as shown in Fig. 1.2 (left). The Origin is placed at its centre of gravity where its weight provides a force W = 12 (-j) N. The supports are positioned at (-1,-1) and (1,-1). Calculate the size of their reaction fores R₁ and R₂ respectively.
   By symmetry, R₁ = R₂ = 6j N

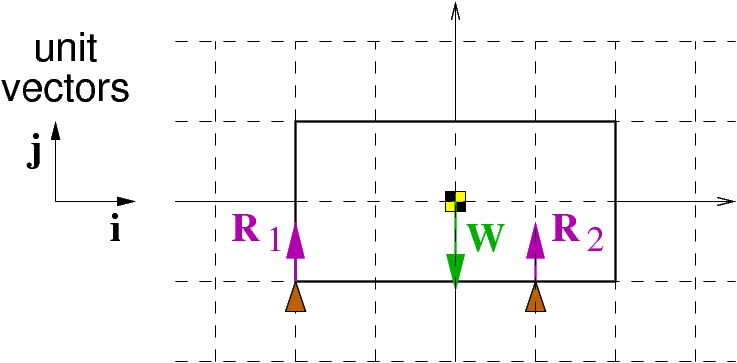
Fig. 1.2: Box with symmetric (left), and asymmetric (right) mounts.




2. Considering moments about O, find the new reaction forces when the first support is re-located at (-2,-1), as in Fig. 1.2 (right).
   Vertical forces, R₁ + R₂ = 12j N
   Moment about the CoG, M = (R₂ -2R₁)k = 0 Nm
   ⇒   R₁ = 4j N and R₂ = 8j N
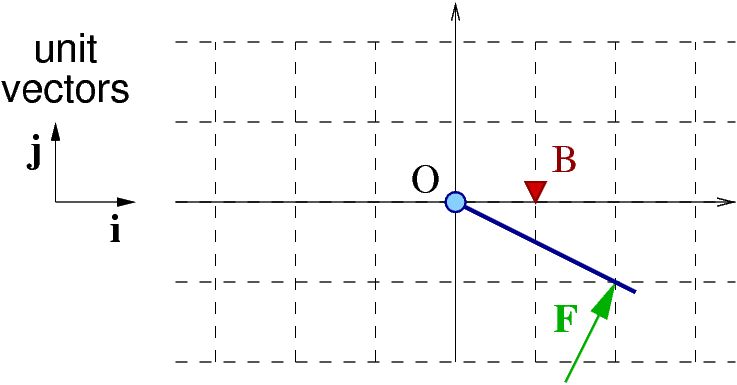
3. A force F = 3 i−6 j N is applied to the handle of a spanner at r = (2,-1), as shown in Fig. 1.3.
   1. What is the magnitude of F?
      F = √(3² + 6²)
   2. What is the length of r?
      r = √(2² + 1²)
   3. What is the angle between the handle of the spanner and F's line of action?
      90° (by gradients of tan^-1)
   4. What is the size of the torque applied to the nut at (0,0)?
      T = rF
   5. What is the direction of the equivalent torque vector?
      k (out of the page/screen)

Fig. 1.3: Force applied to a spanner to produce a torque.




4. The spanner turns and undoes the nut so that it no longer provides any rotational resistance, but still acts as a pivot. The force F maintains the same point of application relative to the spanner. The handle now contacts a bar B located at (1,0). Calculate the magnitude of the vertical force on the bar
   1. if F remains constant
      R_B = 6 × √(2² + 1²)
   2. if F rotates with the spanner, keeping the same magnitude and relative angle.

Note on cross products of unit vectors:	i × j = k	j × k = i	k × i = j
	j × i = −k	k × j = −i	i × k = −j